定义
桶排序是计数排序的升级版。它利用了函数的映射关系,高效与否的关键就在于这个映射函数的确定。
桶排序 (Bucket sort)的工作的原理:假设输入数据服从均匀分布,将数据分到有限数量的桶里,每个桶再分别排序(有可能再使用别的排序算法或是以递归方式继续使用桶排序进行排序)
算法描述
- 人为设置一个BucketSize,作为每个桶所能放置多少个不同数值(例如当BucketSize==5时,该桶可以存放{1,2,3,4,5}这几种数字,但是容量不限,即可以存放100个3);
- 遍历输入数据,并且把数据一个一个放到对应的桶里去;
- 对每个不是空的桶进行排序,可以使用其它排序方法,也可以递归使用桶排序;
- 从不是空的桶里把排好序的数据拼接起来。
注意,如果递归使用桶排序为各个桶排序,则当桶数量为1时要手动减小BucketSize增加下一循环桶的数量,否则会陷入死循环,导致内存溢出。
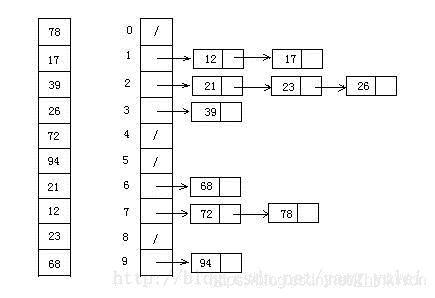
图片演示
代码实现
1
2
3
4
5
6
7
| public static void main(String[] args) {
int[] array = {3, 44, 38, 5, 47, 15, 36, 26, 27, 2, 46, 4, 19, 50, 48};
bucketSort(array);
System.out.println(Arrays.toString(array));
}
|
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
44
45
46
47
48
49
50
51
52
53
54
55
|
public static void bucketSort(int[] array) {
if (array == null || array.length <= 1) {
return;
}
int length = array.length;
LinkedList<Integer>[] bucket = (LinkedList<Integer>[]) new LinkedList[length];
int maxValue = Arrays.stream(array).max().getAsInt();
for (int i = 0; i < array.length; i++) {
int index = toBucketIndex(array[i], maxValue, length);
if (bucket[index] == null) {
bucket[index] = new LinkedList<>();
}
bucket[index].add(array[i]);
}
List<Integer> temp = new ArrayList<>();
for (int i = 0; i < length; i++) {
if (bucket[i] != null) {
Collections.sort(bucket[i]);
temp.addAll(bucket[i]);
}
}
for (int i = 0; i < length; i++) {
array[i] = temp.get(i);
}
}
private static int toBucketIndex(int value, int maxValue, int length) {
return (value * length) / (maxValue + 1);
}
|
算法分析
桶排序最好情况下使用线性时间O(n),桶排序的时间复杂度,取决与对各个桶之间数据进行排序的时间复杂度,因为其它部分的时间复杂度都为O(n)。很显然,桶划分的越小,各个桶之间的数据越少,排序所用的时间也会越少。但相应的空间消耗就会增大。
最佳情况:$T(n) = O(n+k)$
最差情况:$T(n) = O(n+k)$
平均情况:$T(n) = O(n^2)$
